Area Of A Trapezium – Different Ways To Calculate The Area

Finding the area of various shapes like trapeziums may sound difficult in the start due to their forms. However, all you need is the right formula to calculate the area of a trapezium. The area covers a dimensional space that it occupies.

In this article, we’ll define what a trapezium is and show you different ways to find the area.

What Is A Trapezium?

A trapezium is a quadrilateral with a set of parallel sides. The quadrilateral has four parts, but if it has a pair of parallel sides, it’s a trapezoid. However, if both pairs of sides are parallel, it’s a parallelogram.

Some of a trapezium’s properties include:

What Is An Isosceles Trapezoid?

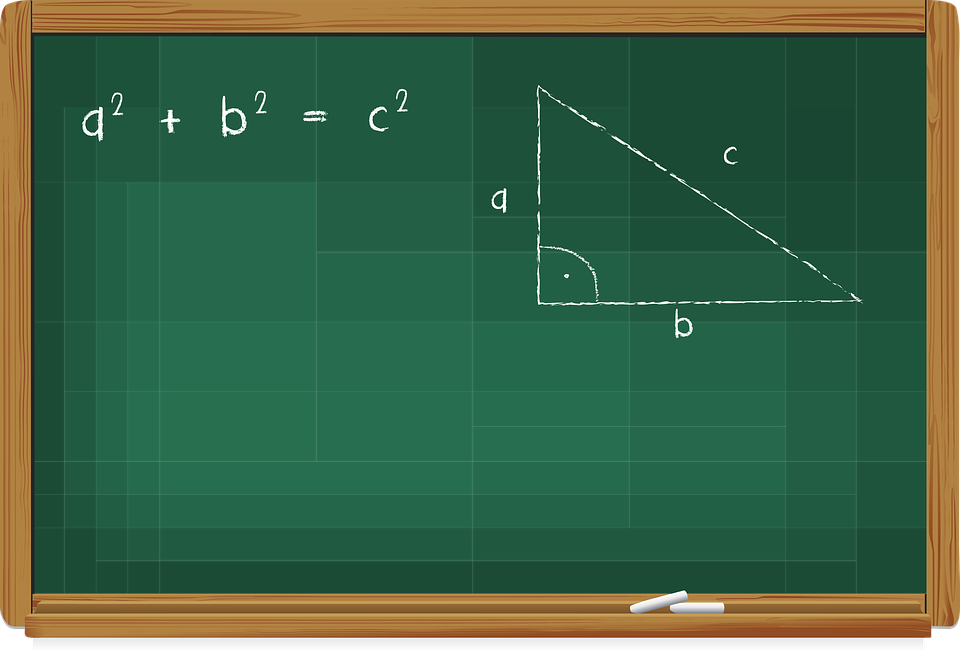
An Isosceles trapezoid is a unique trapezium with equal length legs, with the legs being the non-parallel sides. Some properties of isosceles trapezoid include:

What Is The Origin Of The Word Trapezium?

Euclid wrote his geometry work about 300 B.C. in his book; he did use the word trapezion, as the name of a four-sided figure that wasn’t a rhomboid, rectangle or a rhombus. The name later got a Latin translation to now the trapezium.

Later, Proclus a geometer incorporated specific refinements to Euclid’s work, which have now become geometry basics. Proclus came up with a new meaning of a trapezium, where he referred to it as any four-sided figure with two parallel sides.

The geometer later came up with a new term, trapezoid which denotes a four-sided figure with no two parallel sides. However, in 1795, an English mathematician, Charles Hutton got the descriptions reversed. The US still follows the Hutton’s definition although the British have reverted to Proclus original meaning.

How To Calculate The Area Of A Trapezium

For you to calculate the area of a trapezium, you need to have a formula. Find the length of the nonparallel side, the height of the trapezoid, and the length of one base. The height of a trapezoid is the diagonal line that extends from one corner to another opposite edge.

The basic formula is (B1+B2) x1/2x (H)

Also, you can get the area of a trapezoid by dividing it into two triangles and a rectangle. At this point, you’ll need to calculate the area of both triangles and find the area of the rectangle. Sum these two to get the area of the trapezoid.

The formula for calculating the area of the triangle will be ½ (b-a) h, where b is the length minus the height, and h is the height of the triangle.

The formula for creating the area of the rectangle is ah, where a is the length of the triangle and h is the height.

For example, if you have the height of the trapezium is 8 cm, the lower height 14 cm, and its height 4 cm, you’ll have a rectangle with a length of 8 cm and a height of 4 cm.

The triangle will have 6 cm length when you subtract 14cm from 8 cm. The height of the triangle remains 4 cm.

Now to calculate the area of the trapezium, you’ll need the area of both triangles and the area of the rectangle.

The area of the triangle= (6×4)/2=12

The area of the rectangle= (8×4) =32

Add both these areas to get 44cm2 to get the area of a trapezium.

Also, you can use the previous formula of ½ (b1+b2) h ½ (14+8) x 4=44cm2

What If You Have The Median?

You can find the area of a trapezoid if you have
the median. The median is the line segment that links the midpoints of the
non-parallel sides. Also, the median is the average of two parallel sides.

When you have the median and the height, you can
get the area of a trapezoid using the formula:

A=mh where h is the height and m the median.

Finding The Area Of A Trapezium Without The length Of One  Parallel Side

In some cases, you may only have the length of the diagonal, length of the diagonal, height of the trapezoid, and one nonparallel side. You now need to calculate the area of the trapezoid. Fortunately, you can calculate the area despite having only one nonparallel side.

All you need is to find the length of the base, the height, and the distance of the given nonparallel side. For example, if you
have a trapezoid with 4 inches height, a nonparallel side of 5 inches, and the base equal to 6 inches, you need to identify the length of the other side.

If the trapezoid has 8 inches of diagonal, use the Pythagorean Theorem to find the length of the unknown side.

The formula is (a x 2) + (b x 2) = (c x 2)

The letters a and b represent the two other sides, and c is the hypotenuse.

With the trapezium, you’ll have two triangles. The total of the two unknown sides of the triangles is the length of the hidden side. Use the Pythagorean formula to find the unknown sides.

The second triangle will have a length of 6 inches and the first triangle a length of 3 inches. Add both inches to get the
length of the unknown base as 9 inches.

To now, calculate the area of a trapezoid, ½ (b1 + b2) h = ½ (6 + 9) 4 = 30 inches.

Finding the Area of a Trapezium without Parallel Sides

Sometimes you may need to find the area of a trapezium that doesn’t have parallel sides. To get started, you’ll need to divide the trapezium into two triangles.

The next step is to get the values for an angle of the side, one side that you know, and the other side indicated. You could name the sides a, b, and c. Each triangle will have all the sides above.

To calculate the areas of the triangles, use the formula

A=be/2 (Sin x a)

Put in the values of a, b, and c into the formula to calculate the area of both triangles. Add the areas for the triangles to get the area of a trapezium without parallel sides.

Calculating Missing Values

You may need to figure out the base or the height when you have the area. Here’s how you can get the missing values.

How To Calculate The Height When You Have The Area

While sometimes you can calculate the area using a standard formula, you may be required to figure out the height when given the area. To do this, you’ll need to transpose the conventional method.

You can use algebra to figure out the formula. For example, to get the height, you can use the formula:

H=2A/ (a + b)

Where A is the area and a, b two bases.

To get the value of the base, you need the height, area, and two bases. Rearrange the main formula to get the base.

The formula will be:

B=2A/ (h)-a where A is the area of the trapezium and a is one side of the trapezium.

Applications Of Trapeziums

In biology, you’ll find terms like trapeziform or trapezoidal which refer to specific organs or forms. You can also find the term in architecture as it refers to buildings, windows, symmetrical doors with a broad base and tapering to the top.

The windows on A-frame gables resemble trapezoids with the bottom sides horizontal and the parallel sides running from left to right.

When looking at Egyptian style of building, you’ll notice most of their properties have an isosceles trapezoid shape with straight sides and angular corners. The Inca has this standard style for their windows and doors.

Truss bridges have several trapezoids that connect the base of the bridge to the overhead structure. The aluminum or steel supports create adjacent trapezoids that have parallel sides, which form the bottom and top of the bridge sides.

In Geometry, you’ll find out that the crossed ladders problem is the method of finding the distance between the parallel sides of the right trapezoid when you have the distance from the vertical; base to the slanting intersection and the diagonal lengths.

Most handbags have two trapezoids as the larger sides of the purse. You’ll notice that the top and bottom are parallel, although the top edge is shorter than the one at the bottom.

Final Thoughts

Calculating the area of a trapezium isn’t complicated. All you need is to use the above formula. Alternatively, you can divide the trapezium into two triangles and a rectangle. Calculate the area of the triangles and add this to the area of the rectangle.

Also, you can use trigonometric functions like tangent, cosine, and sine to find the missing sides of either of the triangles. These functions also work when you know the angles of the trapezium.