Trigonometry plays an important role in preparing you for calculus. Learning the right concepts of trig, and understanding them well can help you succeed in calculus. Learn more about how to find a reference angle.
Whether you’re enrolled in a trigonometry or calculus course, both studies have an important relationship. Trigonometry, like algebra, is a common class to take before you move on to calculus. While you might have great success in calculus without experience in trig, it can help you understand many difficult concepts more easily.
In this article, we will discuss how to find a reference angle and discuss some of the basics of trigonometry, just in case it’s been a while since you’ve taken trig or you’re unfamiliar with some of the concepts you learn in trigonometry.
A Quick Look At Trigonometry
Before we can show you how to find a reference angle, you should understand the basics of angles and where they appear on a graph. Trigonometry is the study of angles and how they are used as a means of indirect measurement, especially when distance can be difficult to measure directly.
While trigonometry can often be an overwhelming course in mathematics, there are several opportunities to use the skills you learn in real life applications. Surveyors, engineers, and even architects use angles to measure distances and ensure that measurements are accurate.
Let’s take a quick look at angles and brush up on some of the basics. A ray, which is a line that has a defined endpoint also extends endlessly in one direction. The endpoint of the ray is placed at the origin of an x and y-axis system; the ray lies on the positive x-axis, but you can rotate the ray.
When using the x and y-axis system, rotations that go counterclockwise are positive while clockwise rotations are negative.
If you’re already familiar with angles and graphing, you might remember that an x and y-axis system is divided into four quadrants on the Cartesian plane, which are simply numbered one through 4. The location of the quadrants are as follows:
Quadrant one is located at the top right, and both x and y points are positive. Moving in a counterclockwise rotation, quadrants 2, 3, and 4 continue (with 4 located beneath quadrant one with negative points on the y-axis and positive points on x-axis).
The angle is measured on the ray’s starting position, which is along the positive x-axis (also known as the initial side) and ending at its terminal side.
Before we move on to discussing reference angles, let’s do a little refresher on the types of angles:
- A straight angle is 180°
- A right angle is 90°
- Angles between 90 and 180° are known as obtuse
- Angles between 0 and 90° are acute
There are many other aspects to explore in Trigonometry, but it is most important to discuss things like quadrants as they play an integral role in finding a reference angle.
Finding A Reference Angle
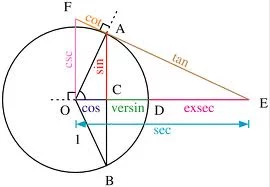
Before we tell you how to find a reference angle, it’s important to define what a reference angle is first. A reference angle is a positive acute angle, which as we just mentioned above means that is between 0 and 90°; a reference angle is never any other type of angle.
Reference angles are found inside of the reference triangle and close to the origin, which is also the vertex. The positive acute angle is between the x-axis and the terminal side.
Before we discuss each quadrant, it’s important to remember that they are ordered from one to four in a counterclockwise direction. You can still find the reference angle is you rotate negatively or if your angles are more than 360° (in our examples we will only find the reference angles for degrees between 0 and 360.
To help you find the reference angle more easily, it’s important to keep the following information on hand. As you practice with more examples, you may not need to reference this table. We will look at each quadrant, the measure of the angle theta (which is used to represent an angle) or ?, and the measure of the reference angle.
Quadrant I has a measure of angle theta is 0° to 90° and the measure of reference angle (R) is theta (?) or R=?.
Quadrant II has a measure of angle theta is 90° to 180°, and the measure of reference angle is 180° minus ? or R=180°-?.
Quadrant III has a measure of angle theta is 180° to 270°, and the measure of reference angle is ? minus 180° or R=?-180°.
Quadrant IV has a measure of angle theta is 270° to 360° and the measure of reference angle is 360° minus ? or R=360°-?.
Considering the information we gave you for each quadrant, it’s time to take a look at some examples and try to find the reference angle when we know what ? equals.
To find the reference angle, sketch out the angle on a graph. Drop a perpendicular to the x-axis to form a triangle.
Example 1:
If ?=135°. You can start by graphing your picture, and you will notice that 135° is in Q II. To find out the reference angle, simply use the quadrant II formula from above. 180-135=45. The reference angle, when ?=135°, is 45°. This is correct because it’s acute and positive.
Remember, the reference angle can never be negative or more than 90°. If your answer is negative more than 90°, you need to look at your problem and start over.
Example 2:
If ?=32°. 32° is in Q I. To find out the reference angle, simply use the quadrant I formula from above. Q I is easy as ?=?. The reference angle of 32° remains unchanged and is 32°.
Example 3:
If ?=329°, 329° is in Q IV. Looking at the formula, 360-329=31. The reference angle, when ?=329°, is 31°.
Example 4:
If ?=251°, 251° is in Q III. Use the quadrant III formula from above to find the reference angle, 251-180=71. The reference angle, when ?=251°, is 71°.
Example 5:
In our next example, we take a look at negative thetas. Since we know that a reference angle must be positive, it may be confusing to put a negative theta in the formula. One of the key differences between negative and positive thetas is that you should rotate clockwise rather than counterclockwise when searching for the correct quadrant.
Remember, your reference angle will not be negative. You should only use the negative number to help you determine which quadrant you’re in.
If ?=-150°, -150° is in Q III. It’s important to note that even though you the negative theta is in Q III, you cannot use the formula as your result will be negative. When finding the reference angle, you will treat the theta as if it is positive. The formula you will use will be Q II (rather than Q III).
So, 180-150=30. The reference angle, when ?=-150°, is 30°.
Example 6:
Let’s try finding the reference angle using another negative theta. If ?=-20°, -20° is in Q IV. When we look at the Q IV formula, it’s 360°- ?. If we followed the formula as is, we would still end up with a negative reference angle. So ignoring the negative sign, ?=20°, which puts it in Q I and the reference angle is 20° since ?=?.
Example 7:
At times, you may be asked to find the reference angle for thetas that are more than 360°. Let’s find the reference angle when the theta is 960°. A 960° angle is the equivalent to a 240° angle. You can find this out by subtracting 360° from 960° two times.
Since ?=240°, you will use the formula from Q III. 240-180=60. The reference angle, when ?=960°, is 60°.
Final Thoughts

Finding the reference angle is often considered as the “fast track” to finding the angle of a triangle. We gave you the basics of the reference angle, but as you progress in your math course, you’re likely to use the reference angle to find the sine, cosine, and tangent of the non-acute angles.
Like all new material you learn in a math course, memorizing can be helpful, but it may be more beneficial to practice until you can find the reference angle without referring to the quadrant formula table.
As with any new concept, you can expect some problems to be easier than others. Allow yourself to try out all kinds of reference angle examples, which include negative numbers and over 360°.